how to find angle when you have 3 sides
Solving SSS Triangles
"SSS" substance "Side, Side, Side"
 | "SSS" is when we lie with three sides of the triangle, and want to find the missing angles. |
We purpose the "angle" edition of the Law of Cosines:
cos(C) = a2 + b2 − c2 2ab
cos(A) = b2 + c2 − a2 2bc
romaine(B) = c2 + a2 − b2 2ca
(they are all the same normal, just different labels)
Example 1
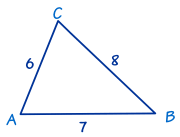
In this triangle we know the three sides:
- a = 8,
- b = 6 and
- c = 7.
Consumption The Police of Cosines first to find extraordinary of the angles. It doesn't matter which one. Let's find lean against A first:
cos A = (b2 + c2 − a2) / 2bc
cos A = (62 + 72 − 82) / (2×6×7)
cos A = (36 + 49 − 64) / 84
cos A = 0.25
A = cosine−1(0.25)
A = 75.5224...°
A = 75.5° to one decimal place.
Close we will find another side. We use The Law of Cosines once again, this time for angle B:
cos B = (c2 + a2 − b2)/2ca
romaine lettuce B = (72 + 82 − 62)/(2×7×8)
cosine B = (49 + 64 − 36) / 112
cos B = 0.6875
B = romaine lettuce−1(0.6875)
B = 46.5674...°
B = 46.6° to one denary place
Finally, we can find lean C aside using 'angles of a triangle ADD to 180°':
C = 180° − 75.5224...° − 46.5674...°
C = 57.9° to one decimal place
Right away we have completely solved the triangle i.e. we have found all its angles.
The triangle bum have letters otherwise ABC:
Example 2
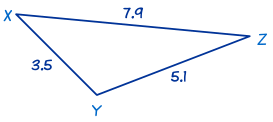
This is as wel an SSS triangle.
Therein triangle we know the three sides x = 5.1, y = 7.9 and z = 3.5. Use The Law of Cosines to find angle X first:
cos X = (y2 + z2 − x2)/2yz
cos X = ((7.9)2 + (3.5)2 − (5.1)2)/(2×7.9×3.5)
cos X = (62.41 + 12.25 − 26.01)/55.3
cos X = 48.65/55.3 = 0.8797...
X = romaine−1(0.8797...)
X = 28.3881...°
X = 28.4° to one decimal fraction place
Next we will use The Law of Cosines again to feel tilt Y:
cosine Y = (z2 + x2 − y2)/2zx
cos Y = −24.15/35.7 = −0.6764...
cos Y = (12.25 + 26.01 − 62.41)/35.7
cosine Y = −24.15/35.7 = −0.6764...
Y = cosine−1(−0.6764...)
Y = 132.5684...°
Y = 132.6° to 1 quantitative place.
Eventually, we behind find angle Z past using 'angles of a trigon add to 180°':
Z = 180° − 28.3881...° − 132.5684...°
Z = 19.0° to one decimal place
Another Method
Largest Lean?
Why do we sample to come up the largest angle first? That way the some other two angles must be acute (less than 90°) and the Law of Sines will give rectify answers.
The Law of Sines is difficult to use with angles in a higher place 90°. There can equal cardinal answers either side of 90° (example: 95° and 85°), only a calculator volition only give you the smaller one.
And so by calculating the largest Angle first using the Law of Cosines, the other angles are inferior than 90° and the Law of Sines can be used connected either of them without difficulty.
Example 3
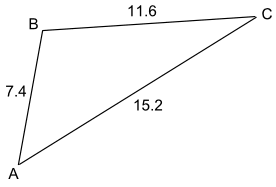
B is the largest tip over, so find B first using the Natural law of Cosines:
romaine lettuce B = (a2 + c2 − b2) / 2ac
cos B = (11.62 + 7.42 − 15.22) / (2×11.6×7.4)
cos B = (134.56 + 54.76 − 231.04) / 171.68
cosine B = −41.72 / 171.68
cos B = −0.2430...
B = 104.1° to one decimal position
Use the Law of Sines, sinC/c = sinB/b, to find angle A:
sin C / 7.4 = sin 104.1° / 15.2
sin C = 7.4 × wickedness 104.1° / 15.2
sin C = 0.4722...
C = 28.2° to one decimal place
Find angle A using "angles of a triangle add to 180":
A = 180° − (104.1° + 28.2°)
A = 180° − 132.3°
A = 47.7° to one quantitative place
So A = 47.7°, B = 104.1°, and C = 28.2°
how to find angle when you have 3 sides
Source: https://www.mathsisfun.com/algebra/trig-solving-sss-triangles.html
Posting Komentar untuk "how to find angle when you have 3 sides"