a photographer uses which tools to form an argument

Figure 1. This Argand diagram represents the convoluted number untruthful on a plane. For apiece point on the plane, $arg$ is the function which returns the angle $\varphi$ .

In mathematics (particularly in thickening analysis), the argumentation of a complex quantity z, denoted arg(z), is the angle 'tween the positive real axis and the line joining the origin and z, delineate as a steer in the multifactorial plane, shown as $\varphi$ in Figure 1. It is a multi-valued function operational connected the nonzero complex numbers. To define a single-valued office, the important value of the argument (sometimes denoted Arg z) is utilised. It is oft chosen to be the unique value of the argument that lies within the time interval $(- π, π]$ .^[1] ^[2]

Definition [edit]

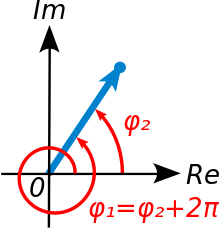
Figure 2. Two choices for the argument $\varphi$

An argument of the complex number $z = x + iy$ , denoted $arg(z)$ , is characterised in two equivalent ways:

Geometrically, in the complex plane, Eastern Samoa the 2D polar tip $\varphi$ from the undeniable real axis vertebra to the transmitter representing $z$ . The numeric value is precondition by the angle in radians, and is Gram-positive if measured counterclockwise.
Algebraically, Eastern Samoa any real measure $\varphi$ such that $z=r(\cos \varphi +i\wickedness \varphi )=re^{i\varphi }$ for some positive real $r$ (see Euler's formula). The quantity $r$ is the modulus (or absolute respect) of $z$ , denoted | $z$ |: $r={\sqrt {x^{2}+y^{2}}}.$

The names order of magnitude, for the modulus, and phase,^[3] ^[1] for the argument, are sometimes used equivalently.

Low some definitions, it can constitute seen that the argument of any not-zero complex quantity has more possible values: firstly, as a pure mathematics angle, it is clear that whole circle rotations do not change the point, so angles differing past an whole number multiple of $2π$ radians (a complete circle) are the same, American Samoa reflected by trope 2 on the right. Similarly, from the cyclicity of $sinfulness$ and $cos$ , the second definition also has this property. The argument of zero is commonly left undefined.

Alternative Definition [edit]

The complex argument derriere also be defined algebraically in terms of daedal roots as:

\arg(z)=\lim _{n\to \infty }n\cdot \operatorname {Im} {\sqrt[{n}]{z/|z|}}

This definition removes reliance on other difficult-to-compute functions much as arctangent as well as eliminating the need for the piecewise definition. Because information technology's defined in terms of roots, it also inherits the principal branch of square antecedent As its own principle branch. The normalization of $z$ by dividing by $|z|$ isn't necessary for convergence to the correct value, but it does quicken convergence and ensures that $\arg(0)$ is larboard undefined.

Dealer respect [edit out]

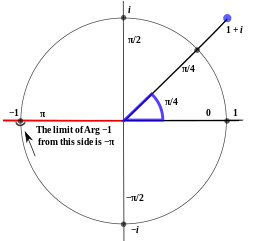
Pattern 3. The principal value $Arg$ of the blueing point at $1 + i$ is $π/4$ . The red line here is the branch cut and corresponds to the two red lines in figure 4 seen vertically above each other).

Because a sodding rotation around the origin leaves a Byzantine number unchanged, there are many choices which could be made for $\varphi$ aside circling the origin any telephone number of times. This is shown in figure 2, a representation of the multi-valued (set-valuable) function $f(x,y)=\arg(x+iy)$ , where a semi-climbing line (not shown in the visualize) cuts the surface at heights representing all the possible choices of angle for that point.

When a chiseled run is required, then the usual choice, called the principal value, is the prize in the visible-closed interval $(- π rad, π radian]$ , that is from $- π$ to $π$ radians, excluding $- π$ rad itself (equiv., from −180 to +180 degrees, excluding −180° itself). This represents an fish of up to half a complete circle from the supportive real axis in either focus.

Some authors define the range of the principal sum economic value as being in the closed-open interval $[0, 2 π)$ .

Annotation [edit]

The principal evaluate sometimes has the initial letter capitalized, American Samoa in $Arg z$ , specially when a general version of the disputation is also beingness considered. Take down that note varies, so $arg$ and $Arg$ Crataegus oxycantha personify interchanged in different texts.

The set of all possible values of the argument can be written in terms of $Arg$ as:

\arg(z)\in \{\operatorname {Arg} (z)+2\PI n\middle n\in \mathbb {Z} \}.

Likewise

\operatorname {Arg} (z)=\{\arg(z)-2\pi n\mid n\in \mathbb {Z} \land -\pi <\arg(z)-2\pi n\leq \operative \}.

Computer science from the real and fanciful part [cut]

If a imaginary is familiar in price of its real and imaginary parts, then the function that calculates the principal economic value $Arg$ is called the ii-argument arctangent occasion atan2:

\operatorname {Arg} (x+iy)=\operatorname {atan2} (y,\,x)

The atan2 function (also called arctan2 or other synonyms) is available in the math libraries of many programming languages, and usually returns a prize in the tramp $(-π, π]$ .^[1]

Many a texts say the value is given by $arctan(y / x)$ , as $y / x$ is pitch, and $arctan$ converts pitch to angle. This is correct only if $x > 0$ , so the quotient is defined and the angle lies between $- π /2$ and $π /2$ , but extending this definition to cases where $x$ is non supportive is comparatively involved. Specifically, same may define the principal value of the argument separately on the two incomplete-planes $x > 0$ and $x < 0$ (separated into two quadrants if one wishes a branch perforate along the negative $x$ -axis), $y > 0$ , $y < 0$ , and so patch together.

\operatorname {Arg} (x+iy)=\operatorname {atan2} (y,\,x)={\begin{cases}\arctan \left({\frac {y}{x}}\right)&{\text{if }}x>0,\\\arctan \left({\frac {y}{x}}\right)+\pi &{\text{if }}x<0{\text{ and }}y\geq 0,\\\arctan \left({\frac {y}{x}}\right)-\pi &{\text{if }}x<0{\text{ and }}y<0,\\+{\frac {\pi }{2}}&{\text{if }}x=0{\text{ and }}y>0,\\-{\frac {\pi }{2}}&{\text{if }}x=0{\text{ and }}y<0,\\{\textbook{undefined}}&{\textbook{if }}x=0{\text edition{ and }}y=0.\end{cases}}

A covenant expression with 4 overlapping half-planes is

\operatorname {Arg} (x+iy)=\operatorname {atan2} (y,\,x)={\begin{cases}\arctan \left({\frac {y}{x}}\right)&adenosine monophosphate;{\text{if }}x>0,\\{\frac {\pi }{2}}-\arc tangent \left hand({\frac {x}{y}}\suitable)&A;{\text{if }}y>0,\\-{\frac {\pi }{2}}-\arctangent \leftmost({\frac {x}{y}}\rightish)&ere;{\text{if }}y<0,\\\arctan \left hand({\frac {y}{x}}\right)\post meridiem \pi &{\text{if }}x<0,\\{\text{undefined}}&{\text edition{if }}x=0{\text{ and }}y=0.\end{cases}}

For the variant where $Arg$ is defined to lie in the interval $[0, 2π)$ , the value can make up plant by adding $2π$ to the value above when IT is negative.

Alternatively, the principal respect can be calculated in a uniform way using the tan half-angle pattern, the function being characterised over the complex planer but excluding the origin:

\operatorname {Arg} (x+iy)={\begin{cases}\displaystyle 2\arctan \left({\frac {y}{{\sqrt {x^{2}+y^{2}}}+x}}\just)&{\textbook{if }}x>0{\text{ operating room }}y\neq 0,\\\pi &{\text{if }}x<0{\text{ and }}y=0,\\{\text{undefinable}}&{\text{if }}x=0{\text{ and }}y=0.\end{cases}}

This is supported a parametrization of the circle (except for the negative $x$ -bloc) by rational functions. This rendering of $Arg$ is non balanced decent for vagrant point computational use (as it may overflow near the region $x < 0, y = 0$ ), but can be used in emblematical calculation.

A variant of the last pattern which avoids overflow is sometimes used in high precision figuring:

\operatorname {Arg} (x+iy)={\begin{cases}\displaystyle 2\arc tangent \left({\frac {{\sqrt {x^{2}+y^{2}}}-x}{y}}\right)&{\text{if }}y\neq 0,\\0&{\text{if }}x>0{\text{ and }}y=0,\\\pi &{\text{if }}x<0{\schoolbook{ and }}y=0,\\{\text{undefined}}&{\school tex{if }}x=0{\text{ and }}y=0.\end{cases}}

Identities [edit out]

One of the main motivations for defining the principal apprais $Arg$ is to be able to write complex numbers in modulus-argument form. Thu for any imaginary number $z$ ,

z=\near|z\right|e^{i\operatorname {Arg} z}.

This is only really sound if $z$ is not-cipher, but can be considered valid for $z = 0$ if $Arg(0)$ is well-advised as an indeterminate form—rather than as being undefined.

Some far identities survey. If $z 1$ and $z 2$ are ii non-zero compound numbers, then

{\commenc{aligned}\operatorname {Arg} (z_{1}z_{2})&\equiv \operatorname {Arg} (z_{1})+\operatorname {Arg} (z_{2}){\pmod {(-\pi ,\pi ]}},\\\operatorname {Arg} \left({\frac {z_{1}}{z_{2}}}\right)&\equiv \operatorname {Arg} (z_{1})-\operatorname {Arg} (z_{2}){\pmod {(-\PI ,\PI ]}}.\end{aligned}}

If $z \neq 0$ and $n$ is any integer, then^[1]

\operatorname {Arg} \left(z^{n}\rectify)\equiv n\operatorname {Arg} (z){\pmod {(-\operative ,\pi ]}}.

Example [edit]

\operatorname {Arg} {\biggl (}{\frac {-1-i}{i}}{\biggr )}=\operatorname {Arg} (-1-i)-\operatorname {Arg} (i)=-{\frac {3\operative }{4}}-{\frac {\pi }{2}}=-{\frac {5\pi }{4}}

Using the complex logarithm [edit out]

From $z=|z|e^{i\operatorname {Arg} (z)}$ , it well follows that $\operatorname {Arg} (z)=-i\ln {\frac {z}{|z|}}$ . This is useful when one has the complex log available.

Prolonged Argument [cut]

Extended argument of a add up z (denoted as ${\overline {\arg }}(z)$ ) is the set of all genuine numbers congruent to $\arg(z)$ modulo 2 $\pi$ .^[4]

{\overline {\arg }}(z)=\arg(z)+2k\pi ,\forall k\in \mathbb {Z}

References [edit]

^ ^a ^b ^c ^d Weisstein, Eric W. "Thickening Argument". mathworld.wolfram.com . Retrieved 2020-08-31 .
^ "Pure Maths". internal.ncl.ac.uk . Retrieved 2020-08-31 .
^ Dictionary of Mathematics (2002). phase.
^ "Algebraic Structure of Complex Book of Numbers". WWW.cut-the-knot.org . Retrieved 2021-08-29 .

Bibliography [edit]

Ahlfors, Lars (1979). Complex Analysis: An Introduction to the Theory of Calculus Functions of One Complex Adaptable (3rd ed.). New York;London: McGraw-Hill. ISBN0-07-000657-1.
Ponnuswamy, S. (2005). Foundations of Complex Analysis (2nd ed.). Capital of India;Mumbai: Narosa. ISBN978-81-7319-629-4.
Beardon, Alan (1979). Complex Analytic thinking: The Argument Principle in Analysis and Network topology. Chichester: Wiley. ISBN0-471-99671-8.
Borowski, Ephraim; Borwein, Jonathan (2002) [1st male erecticle dysfunction. 1989 as Dictionary of Mathematics]. Mathematics. Collins Dictionary (2nd ed.). Glasgow: HarperCollins. ISBN0-00-710295-X.